|
Parallel numerical solution of optimal control problems with instationary diffusion-convection-reaction equations |
partial project A15 of SFB393 Parallele Numerische Simulation für Physik und Kontinuumsmechanik
worked on by: Prof. Dr. Peter Benner, Dipl. Math. Jens Saak
This project
considers boundary value problems with linear partial differetial
equations and boundary control.
previous work:
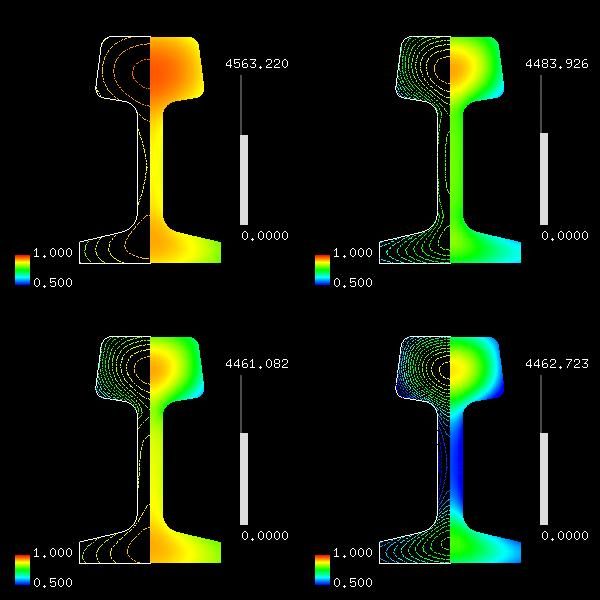
In his diplom thesis Jens Saak worked on the controlled cooling of steel
profiles during production process looking at railprofiles as
an example. It was taken into concern that different
areas of the surface of the material should be cooled
differently intense.
The starting ponit from the view of an engineer was the fact that an equally distributed temperatureprofile on some cross-section of the profile before the last rolling established by selective cooling leads to better mechanical material characteristics of the endproduct than using conventional rolling without selective cooling.
The rising mathematical problem is that
of optimal control using boundary control for a (linearised)
heat-equation. The variational formulation leads to a linear
quadratic optimal control problem in classical formulation
which in contrary to classical problems is formulated in an
infinite dimensional hilbert-space. This problem due to
Gibson 1979 can be solved by a feedback approach.
The Numerical implementation uses fem-semidiscretisation in space. This lead to a lqr-problem for an ordinary differential equation. This equation has Avery large (>1000) dimension which leads to additional difficulties for the numerical treatment.
additional information:
- overview poster for SFB survey (german only)
- diploma thesis by Jens Saak: Effiziente numerische Lösung eines Optimalsteuerungsproblems für die Abkühlung von Stahlprofilen (german only)
- Efficient numerical Solution of the LQR problem for the heat equation; Proc. Appl. Math. Mech., 2004.
- Linear-Quadratic Regulator Design for Optimal Cooling of Steel Profiles.
Our System matrices part of the Oberwolfach Model Reduction Benchmark Collection. They can be found here.